Percentage.

 Percentage SET 01: Q1-Q5

1. Out of two numbers, 65% of the smaller number is equal to 45% of the larger number. If the sum of two numbers is 2574, then what is the value of the larger number?
1
1521
2
1471 
3
1641
4
1419

Solution: 1

Given:

Out of two numbers, 65% of the smaller number is equal to 45% of the larger

number. If the sum of two numbers is 2574

Calculation:

Let the smaller number be ‘x’ and the larger number be ‘y’

From the problem, it is given that

65%x = 45%y

⇒ 13x = 9y

⇒ x = (9/13)y    ----(1)

Given the sum of the numbers = 2574

⇒ (x + y) = 2574     ----(2)

Substituting the value of ‘x’ from Equation 1 in Equation 2, we get

(9/13)y + y = 2574

⇒ (9y + 13y) = 2574 × 13

⇒ 22y = (2574 × 13)

⇒ y = (2574 × 13)/22 = 1521

∴ Value of the larger number is 1521

2. Two numbers are 50% and 75% lesser than a third number. By how much percent is the second number to be enhanced to make it equal to the first number?

1
50
2
25
3
75
4
100

Solution: 4

Let the third number = x

⇒ The first number = x - 50% of x = x/2

⇒ The second number = x - 75% of x = x/4

⇒ The percent by which second number has to be enhanced to make it equal to the first number = [(x/2 - x/4) / (x/4)] × 100 = 100%

(It can also be seen that x/4 is just half of x/2 so it has to be multiplied twice to become the second number which is 100%)

Alternate method

Let, 3rd number be 100

Hence, 2nd number will be 25 and 1st number will be 50

Now, 2nd number should be increased by 25 to make it equal to 1st number

∴ required percentage = 25/25 × 100 = 100%


3. If the price of petrol be raised by 20%, then the percentage by which a car owner must Reduce his consumption so as not to increase his expenditure on petrol is 

1

2

3

4

Solution: 2

Percentage decreases in the consumption of petrol


Detailed solution

Let initially price be 100rs\lt and consumption be 100lt.

Now, increased price = 120% of 100 = 120

New consumption = (100 × 100)/120 = 83.33

Hence, percentage reduction in consumption = [(100 - 83.33)/100] × 100 = 16.67% = 


4. A and B are two fixed points 5 cm apart and C is a point on AB such that AC is 3 cm. If the Length of AC is increased by 6%, the length of CB is decreased by how many % ?

1
6%
2
7%
3
8%
4
9%

Solution: 4

 

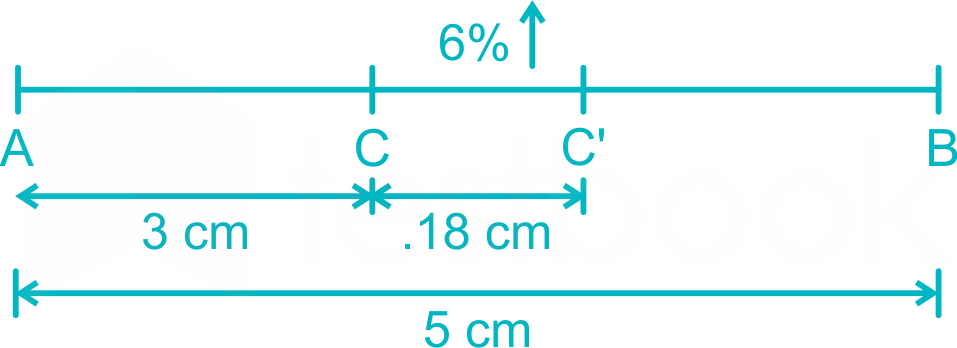
Increase in AC = 6%

Increase in AC 

Decrease in CB = 5 - 3.18 = 1.82 cm

Decrease = 2 - 1.82 = 0.18 cm

So Percentage decrease 


5. A glass of juice contains 5% fruit extract, 25% of pulp and rest of water. Find amount of water that should be added in glass of 450 ml juice to reduce pulp concentration to 15%?

1
226
2
300
3
224
4
223

Solution: 2

Juice of 450 ml contains 5% fruit extract, 25% of pulp.

Amount of pulp = 450 × 25/100 = 112.5 ml

Now, let the amount of water added be ‘x’ ml.

Total volume of juice after adding water = 450 + x

New percentage of pulp = 15%

According to the question

⇒ (450 + x) × 15/100 = 112.5

⇒ (450 + x) = 750

∴ x = 300 ml


 SET 01 : Q1 - Q5

 SET 05 : Q21 - Q25

SET 06 : Q26 - Q30



No comments:

Post a Comment